Aurélie 11/04/07
 

Concours Capes : Etude électrique du rotor du moteur asynchrone 2007

Google

 

Les vecteurs sont écrits en gras et en bleu.

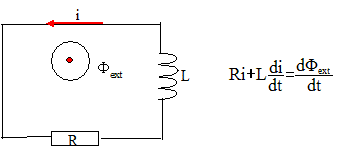
Le rotor du moteur asynchrone est une bobine plate, fermée sur elle même, de surface totale S=Suz, de résistance R, d'inductance L qui peut tourner autour de l'axe (Oz) passant par son centre et perpendiculaire à S. La bobine est solidaire d'un volant de grand moment d'inertie régularisant sa vitesse de rotation WS =wSuz. Le rotor du moteur asynchrone ressent le champ magnétique crée par le stator, de valeur constante B0, tournant autour de l'axe (Oz) à la vitesse angulaire constante WB =wBuz. Le retard de phase initial de la bobine sur le champ tournant est j.

  1. Déterminer l'équation différentielle qui régit le courant i(t) dans la bobine du rotor, en fonction de R, L et de Fext, le flux du champ magnétique extérieur à travers la bobine du rotor.
  2. Représenter dans le plan (Oxy) le champ tournant B et le vecteur surface S en faisant figurer l'angle j à l'instant t.
  3. Exprimer Fext en fonction de t, B0, S, wB, wS et j.
  4. On veut exprimer l'intensité sous la forme i(t) = Imax cos(wt+j-Y). Exprimer Imax en fonction de w, R, L, B0 et S.
  5. Faire de même pour sin Y.
Equation différentielle qui régit le courant i(t) dans la bobine du rotor :

 

Dans le plan (Oxy), représentation du champ tournant B et du vecteur surface S :

 

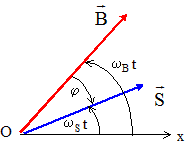
Expression de Fext en fonction de t, B0, S, wB, wS et j.

Fext(t) = B(t) . S(t) avec Bx(t) = -B0sin( wB t ) ; By(t) =B0 cos( wB t )

et Sx(t) = S sin( wS t -j) ; Sy(t) =B0 cos( wS t -j )

Fext(t) = Bx(t)Sx(t) + By(t)Sy(t)

Fext(t) =B0S [-sin( wB t )sin( wS t -j) +cos( wB t )cos( wS t -j )]

Or -sin( wB t )sin( wS t -j) +cos( wB t )cos( wS t -j ) = cos (( wB-wS)t +j)

Fext(t) =B0Scos (( wB-wS)t +j).
 

Expression de Imax en fonction de w, R, L, B0 et S :

L''équation différentielle est résolue par la méthode des nombres complexes ; on pose w = wB-wS

Ri + Ldi/dt =d Fext/dt ; Fex = B0Scos( wt +j) ; i(t) = Imax cos(wt+j-Y).

grandeur physique
i(t)
di/dt
d Fext/dt
nombre complexe associé
Imax exp j(j-Y)
jwImax exp j(j-Y)
jw B0Sexp jj
L'équation différentielle s'écrit alors :

R I max exp j(j-Y) + jLwImaxexp j(j-Y)= jw B0Sexp jj.

(R+jLw)I maxexp j(-Y) = jw B0S

Par suite en égalant les modules [R2+(Lw)2 ] I2 max = (w B0S)2.

Imax= w B0S [R2+(Lw)2 ] -½.

arg [(R+jLw)I max] -Y = arg ( jw B0S)

Y = arg ( jw B0S) - arg [(R+jLw)I max]

arg ( jw B0S) = ½p ; arg [(R+jLw)I max] = tan-1(Lw/R).

Y =½p -arctan(Lw/R).

½p -Y =arctan(Lw/R) ou sin(½p -Y )= cosY =Lw /[R2+(Lw)2 ] -½ .

 
 

retour -menu